Coefficient of Friction Calculator | Friction of 2 Surfaces

The Coefficient of Friction Calculator is an invaluable tool for students, engineers, and professionals working in fields involving mechanics and material sciences. By inputting the normal force and the friction force, this calculator efficiently determines the coefficient of friction between two surfaces.

Alternatively, it can calculate the friction force or normal force when the other variables are known. This calculator simplifies complex calculations involved in understanding the frictional interaction between surfaces, making it a vital resource for practical applications in physics, engineering design, and safety assessments.

Normal Force (N):

Frictional Force (N):

Calculate

Coefficient of Friction:

Friction is a fundamental force in the physical world. It’s what keeps our shoes from slipping on the sidewalk, and our cars from skidding on the road, and it plays a vital role in many engineering applications.

The Coefficient of Friction is a measure that quantifies the resistance to motion between two surfaces in contact. In this article, we will explore the Coefficient of Friction Calculator and the formula behind it.

How to Use the Coefficient of Friction Calculator

To effectively use this calculator:

1. Input Known Variables: Fill in any two of the following fields: normal force (in Newtons), friction force (in Newtons), or the coefficient of friction. Leave the unknown variable blank.
2. Calculate: Click the ‘Calculate’ button to compute the missing value.
3. View the Result: The calculated value (coefficient of friction, normal force, or friction force) will be displayed.
4. Reset for New Calculations: Use the ‘Reset’ button to clear all inputs for a fresh calculation.

Explanation of the Coefficient of Friction

The coefficient of friction is a dimensionless scalar value that describes the ratio of the force of friction between two bodies and the force pressing them together. The formula is:

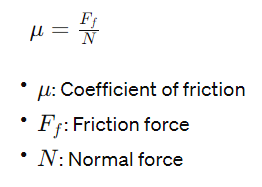
Definition and Background of Friction

Friction is the resistance to motion of one object moving relative to another. It’s a fundamental concept in physics, playing a critical role in areas like mechanics, automotive engineering, and material science.

Step-by-Step Calculation Guide for Coefficient of Friction

1. Determine the Known Variables: Identify the available values among normal force, friction force, and coefficient of friction.
2. Enter the Values: Input the known values in the respective fields.
3. Calculate the Unknown Variable: The calculator will find the missing value based on the input data.

Table of Example Calculations for Coefficient of Friction

Normal Force (N)	Friction Force (N)	Coefficient of Friction	Calculated Value
10	5	–	μ = 0.50
–	20	0.4	N = 50
30	–	0.3	Ff = 9 N

Quick Glossary for Coefficient of Friction

• Normal Force: The force perpendicular to the contact surface.
• Friction Force: The force opposing the relative motion or tendency of such motion of two surfaces in contact.

The Coefficient of Friction Formula

The Coefficient of Friction (μ) is calculated by dividing the Frictional Force (Ff) by the Normal Force (Fn). The formula can be expressed as:

μ = Ff / Fn

Where:

• μ is the coefficient of friction.
• Ff is the frictional force between two surfaces.
• Fn is the normal force, which is the force perpendicular to the contact surfaces.

Using the Coefficient of Friction Calculator

This calculator is a handy tool for determining the coefficient of friction between two surfaces. It requires two inputs:

1. Normal Force (N): This is the force acting perpendicular to the contact surfaces. For example, if you’re calculating the friction between a block and a table, the normal force is the weight of the block, which is the force due to gravity.
2. Frictional Force (N): This is the force that opposes the relative motion of the two surfaces. It’s the force you’re trying to measure or understand.

Calculating the Coefficient of Friction

Once you’ve entered the values for the Normal Force and Frictional Force, click the “Calculate” button.

The calculator will use the formula discussed earlier to determine the coefficient of friction. The result will be displayed in the “Coefficient of Friction” input field.

Significance of the Coefficient of Friction

The coefficient of friction is a crucial parameter in various fields. For example, in engineering, it’s used to design brakes for vehicles, ensure stability in structures, and optimize machinery performance.

In everyday life, it helps us understand how different surfaces interact and how much force is required to move objects.

Conclusion

The Coefficient of Friction Calculator simplifies the process of determining the coefficient of friction between two surfaces.

By understanding this parameter, you can make informed decisions in various applications, from engineering to everyday activities.

FAQ Section

Q: What affects the coefficient of friction between two surfaces? A: Factors include the materials of the surfaces, surface textures, and any substances between them like lubricants.

Q: Can the coefficient of friction be greater than 1? A: Yes, a coefficient greater than 1 indicates a high frictional force relative to the normal force.

Q: Is the coefficient of friction constant for a given pair of surfaces? A: The coefficient of friction is generally considered constant for a specific pair of materials under consistent conditions. However, it can vary with changes in factors like temperature, surface finish, and presence of contaminants.

Q: Does the coefficient of friction depend on the size of the contact area? A: No, the coefficient of friction is independent of the contact area size. It depends primarily on the nature of the materials in contact and their surface properties.

Q: How is the coefficient of friction used in real-world applications? A: It’s crucial in designing mechanical systems, ensuring safety in vehicle design, calculating the necessary force for movement in machinery, and in understanding wear and tear of materials.

By providing these comprehensive insights into the Coefficient of Friction Calculator, users can effectively utilize this tool for educational, professional, or personal applications, deepening their understanding of friction and its implications in various fields.